

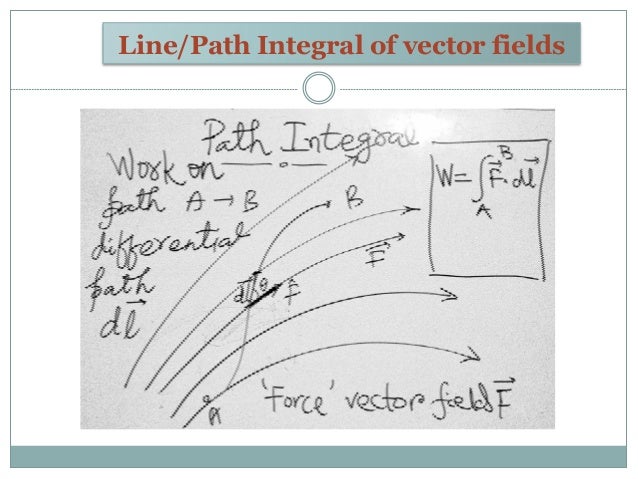
The final result of the dot product process can be: And the rules of (0, 4) are "Destroy your banana growth, and quadruple your orange growth." When applied to something with only bananas, like (3, 0), we're left with nothing. We're mutating the original vector based on the rules of the second. See how we're "applying" and not simply adding? With regular addition, we smush the vectors together: (3,0) + (0, 4) = (3, 4). But (3, 0) had no orange growth to begin with, so the net result is 0 ("Destroy all your fruit, buddy"). (0,4) means "Destroy your bananas, quadruple your oranges"Īpplying (0,4) to (3,0) means "Destroy your banana growth, quadruple your orange growth".(3,0) means "Triple your bananas, destroy your oranges".Now they're not the same type of number: what happens when apply growth (use the dot product) in our "bananas, oranges" universe? Let's say 3 means "triple your bananas" (x-axis) and 4 means "quadruple your oranges" (y-axis). Now, suppose 3 and 4 refer to different dimensions. 3 x 4 = 12 means we get 12x growth in a single dimension. The number 3 is "directional growth" in a single dimension (the x-axis, let's say), and 4 is "directional growth" in that same direction. Let's start simple, and treat 3 x 4 as a dot product: The "this stuff = that stuff" equation just means "Here are two equivalent ways to 'directionally multiply' vectors". Polar perspective: combine magnitudes and angles.Rectangular perspective: combine x and y components.The equation above shows two ways to accomplish this: The goal is to apply one vector to another. The goal is to apply one vector to another.You've seen the dot product equation everywhere:Īnd also the justification: "Well Billy, the Law of Cosines (you remember that, don't you?) says the following calculations are the same, so they are." Not good enough - it doesn't click! Beyond the computation, what does it mean? You've seen the dot product equation everywhere:Īnd also the justification: "Well Billy, the Law of Cosines (you remember that, don't you?) says the following calculations are the same, so they are." Not good enough - it doesn't click! Beyond the computation, what does it mean? With the quaternions (4d complex numbers), the cross product performs the work of rotating one vector around another (another article in the works!).“Multiply” two vectors when only perpendicular cross-terms make a contribution (such as finding torque).Determine if two vectors are orthogonal (checking for a dot product of 0 is likely faster though).Find the signed area spanned by two vectors.Find the direction perpendicular to two given vectors.(Try it: using your right hand, you can see x cross y should point out of the screen). In a computer game, x goes horizontal, y goes vertical, and z goes “into the screen”. The Unity game engine is left-handed, OpenGL (and most math/physics tools) are right-handed.
I never really memorized these rules, I have to think through the interactions. This completed grid is the outer product, which can be separated into the:ĭot product, the interactions between similar dimensions ( x*x, y*y, z*z)Ĭross product, the interactions between different dimensions ( x*y, y*z, z*x, etc.) Taking two vectors, we can write every combination of components in a grid:


 0 kommentar(er)
0 kommentar(er)
